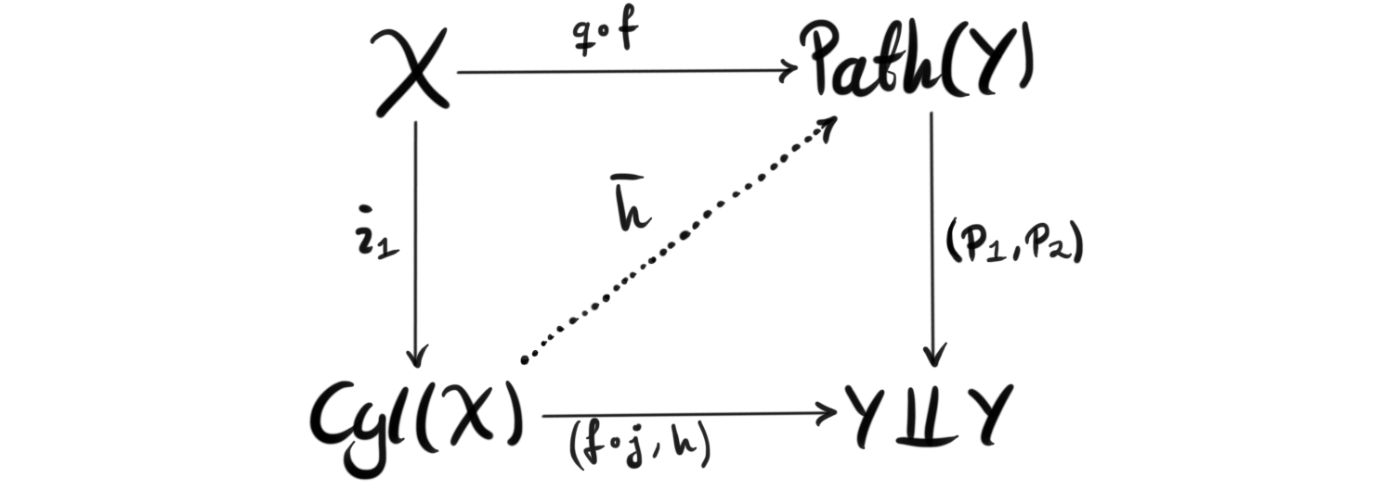
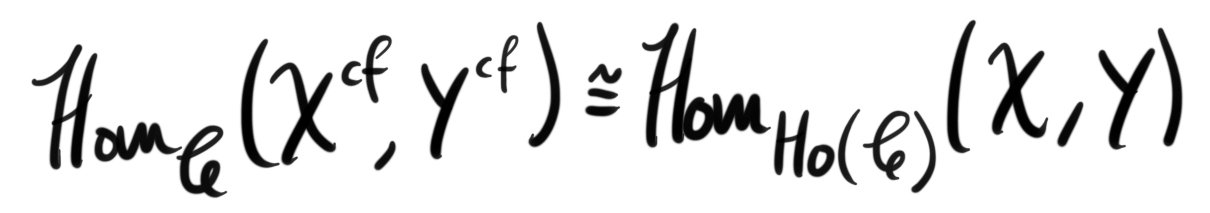The homotopy groups of the spheres. Part 1
Recently I gave a talk about the homotopy groups of spheres, and as usual, I try to collect my thoughts on this blog before (or after) presenting. The homotopy groups of spheres have featured several times on this blog, and we have made some effort into calculating them for some small dimensions. In the talk I wanted to showcase some methods used to calculate these groups, as well as doing some of the “calculations”....